How a Discount for Lack of Marketability (DLOM) is Determined
In a prior chapter of my professional career, I focused on equity security valuations for tax and financial reporting purposes. I led a team of valuation experts who determined the strike price of options granted to employees of up-and-coming technology companies on their way to IPO. For most, that strike price represents the basis (or cost) of an employee’s future wealth (and tax bill). In simple terms, the valuation of these shares in private companies is based on market multiples (or their value divided by a specific operating metric like sales or earnings) of comparable public companies. Once the value is allocated to common shares, the per-share price is a marketable value because it is based on the stock prices of marketable securities (the publicly traded companies we used to compare). Because private company shares are not as marketable (liquid) as public company shares, we need to adjust for this relative lack of marketability. But how?
Enter the Black-Scholes Model
One answer lies in the economic studies of the early 1970s. In 1973, Fisher Black, Myron Scholes, and Robert Merton published “The Pricing of Options and Corporate Liabilities” in the Journal of Political Economy introducing a simple model with five key inputs. While the formula truly has some high-level calculus, I will try to simplify the output and how it is used.
Here are some definitions you will need to understand before we start:
- Current Price – The current per-share value of the stock.
- Dividend Yield – The annual return to an investor in the form of dividends as a % of the stock price.
- Strike Price – The predetermined price.
- Maturity – The amount of time in years until the option expires.
- Risk-free Rate – The rate of a return for a risk-free investment, in this case, a US Government debt instrument at the same maturity term as the put option
- Volatility – The rate at which a security increases or decreases over time.
- Call Option – The option or right to buy (or call) a security at a predetermined price by a predetermined date.
- Put Option – The option or right to sell (or put) a security at a predetermined price by a predetermined date.
How a Put Option Works
Before I dive into how a Discount for Lack of Marketability (DLOM) is determined, I want to examine a real-life example of what marketability is worth. For my example, I’m only going to look at buying a put option. The purchase of a put option assumes that the buyer is a long-term holder looking to protect his/her position in security. I chose a put option because it can be easily compared to an insurance policy.
Facts:
- Stock Owned: Jane owns 100 shares of Microsoft currently priced at $257.24 as of the close of the financial markets on June 10, 2021. This price very close to its 52 weeks high of $263.19 per share.
- Goal: Jane wants to lock in the current price (or close to it) for the next year and is willing to pay for the security of knowing that she can lock in that price.
- Publicly Traded Options: Jane can buy a put option for one year (or with an exercise price of $255.00 in June 2022) at $26.56.
- Options Purchased: Jane purchases 100 put options for $2,656 to lock in the sale of her shares when the option expires in June 2022 for $25,500.
What this Means for Jane
Jane just bought an insurance policy that lets her get the $255.00 per share for her stock. The put option ensures the marketability of her shares but it came at a cost of 10.4% of the value of her shares (or $2,656 divided by $25,500). While she can’t exercise the option until it expires (the terms of a standard put option) she can sell the options if they increase in value before it expires.
That option will increase in value as the price of Microsoft drops because it is worth more to someone to sell shares at a higher price in the future. An example of this concept is the fact that the put option for the same June 2022 date but at a price of $245.00 is selling at $31.93. In simple terms, the price is driven by the supply (people willing to bet that when the option expires in a year the price of Microsoft will be much higher than it is today) and demand (investors like Jane that simply want the protection of believing that the price will be less in a year than it is today).
How this Relates to a DLOM in Valuing Private Company Shares
Let’s replace Jane with Jack and let’s assume that Jack’s investment is 100 shares in Software Widget, Inc., a private company with no publicly traded market for its shares.
Say Jack hires Exit Strategies to value his 100 shares. We value the shares based on market multiples of publicly traded (or “marketable”) companies comparable to Software Widget, Inc. and arrive at a price per share of $50.00 a share. But Jack’s shares aren’t marketable. He can’t call his broker to sell them and certainly can’t buy a put option to protect the value of his shares. Like Jane, he expects the shares to be marketable in a year but unlike Jane, he can’t buy a real insurance policy to lock in that price. Simply put he lacks marketability for the next year but what does this mean to the value we place on his shares?
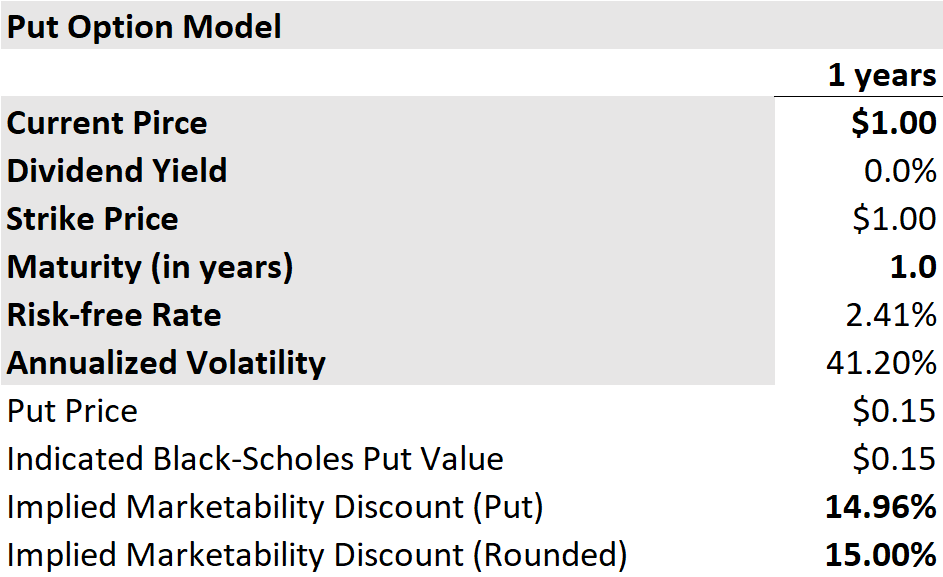
The answer lies in the put option that we discussed above but instead of looking for the price of one on Google Finance, we need to go back to the formula Black and Scholes determined to build up and calculate a hypothetical one. Using the inputs below highlighted in gray, the hypothetical option asks a simple question; what would it cost to buy a hypothetical put option to lock in the price of a security at $1.00 for one year? In the case of the inputs below, the answer is $0.15 or 15.0% of the value of the security. Because it would cost $0.15 per share to lock in the price of $1.00 over a year, the lack of this marketability is the cost of not having this protection (or to Jane’s example, an insurance policy).
So back to Jack. His shares are worth $50.00 per share on a marketable basis but we need to value them on the non-marketable basis of a private company. Therefore, we apply a 15% discount to arrive at our concluded price of $42.50 as detailed below:

This is just one way to determine a DLOM
In determining discounts for lack of marketability, Exit Strategies also considers studies that map actual discounts of restricted stock. The uniqueness of the put option model approach above lies in the inputs and how the discounts change when one of the three key inputs (dividend yield, maturity, and volatility) changes. For example, if we change the term above to 5 years, the discount goes to 28%. That increase makes sense because an “insurance policy” to lock in a price would cost more for 5 years than it would for one.
If you have questions about discounts for lack of marketability or if you would like us to value your private business or equity interest for any purpose, email Joe Orlando at jorlando@exitstrategiesgroup.com.